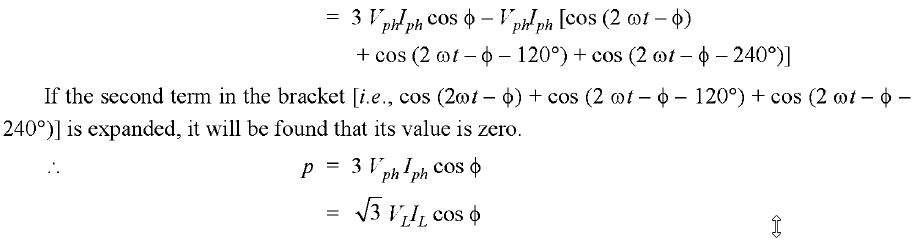Electrical Engineering ⇒ Topic : Constancy of Total Power in Balanced 3-phase System

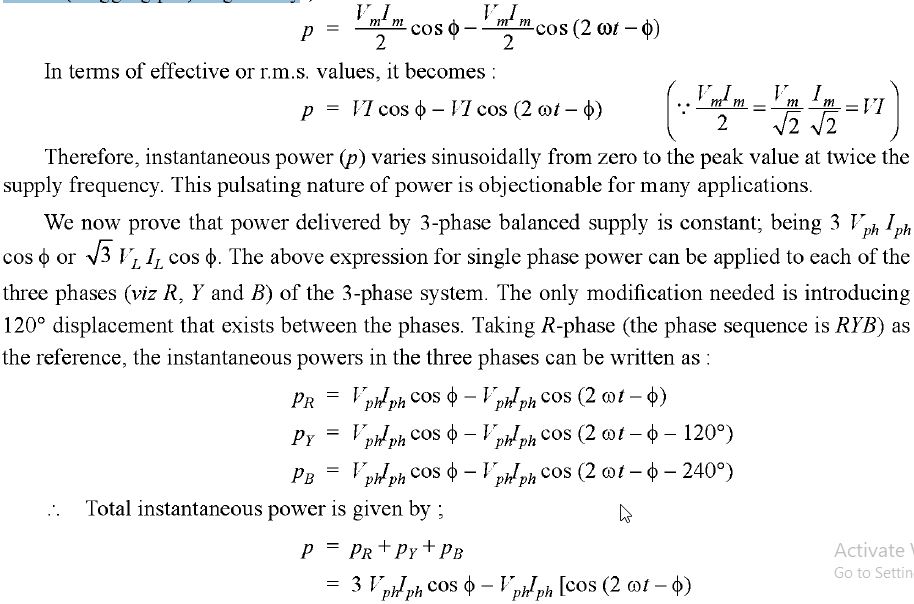
Constancy of Total Power in Balanced 3-phase System

It was shown in chapter  that the instantaneous power p supplied by a single-phase source to a load (*lagging p.f.) is given by

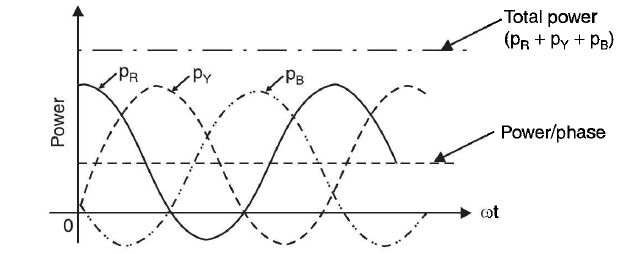
Since phase and line values and power factor for a balanced 3-phase system are fixed, total 3-phase power shall remain constant at all times. Therefore, an important advantage of a 3-phase system over the single-phase system is this constant and smooth power flow into a load such as a 3-phase motor. For this reason, 3-phase motors are used when the load is greater than 3 h.p.Moreover, 3-phase system presents overall economic and operating advantages compared with single-phase system or other polyphase systems. Therefore, the 3-phase system is the most widely used of all the polyphase systems

                                                            figure (a)

Graphical Explanation. Fig. (a) shows the graphical explanation for the constancy of total power in a 3-phase balanced system. Thus p_R represents the power supplied to phase R. The power graph for phase Y lags by 120° behind p_R and is represented by p_Y,. Similarly, graph p_B represents the power supplied to phase B. If we sum up the three curves point by point, it will be seen that total power is constant as shown by the dotted horizontal line in Fig. (a). Thus, although the three component powers fluctuate, the total power is constant. This is due to the phase difference between the fluctuations of the components. Due to the constant power supplied by a balanced 3-phase system, large loads are always driven by 3-phase motors rather than single phase motors