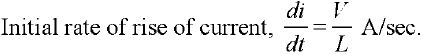Electrical Engineering ⇒ Topic : Rise of Current in an Inductive Circuit

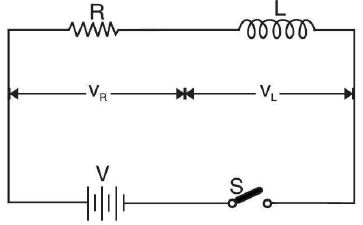
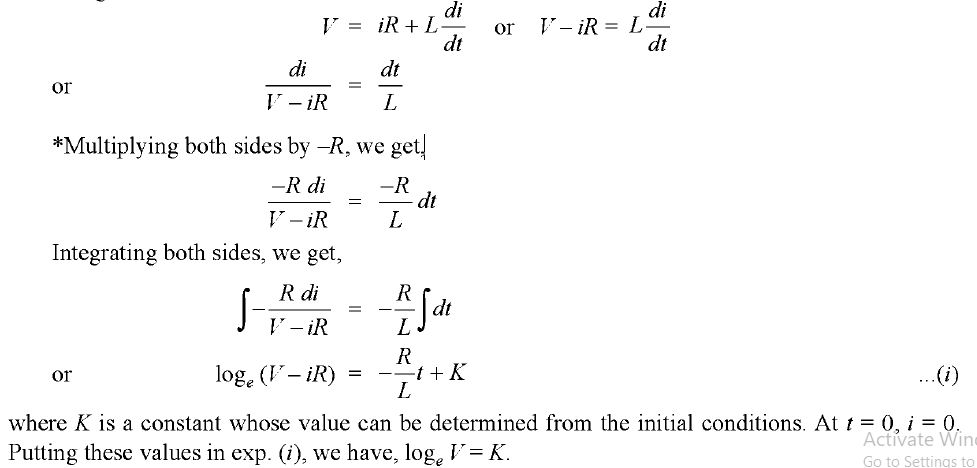
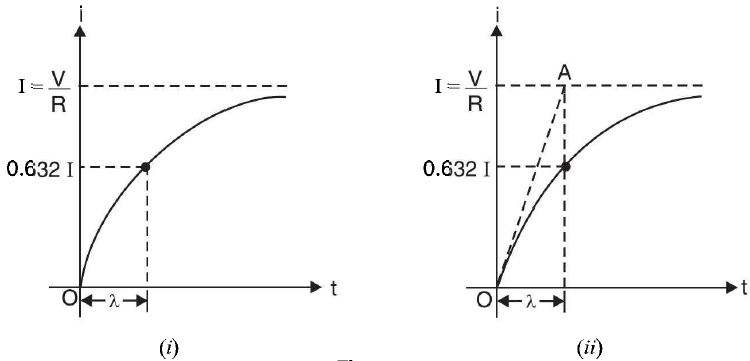
Rise of Current in an Inductive Circuit

Consider an inductive circuit shown in Fig. (a). When switch S is closed, the current rises from zero to the final value I (= r /R) in a small time t. Suppose at any instant, the current is i and is increasing at the rate of di/dt. Then

                                                              figure (a)

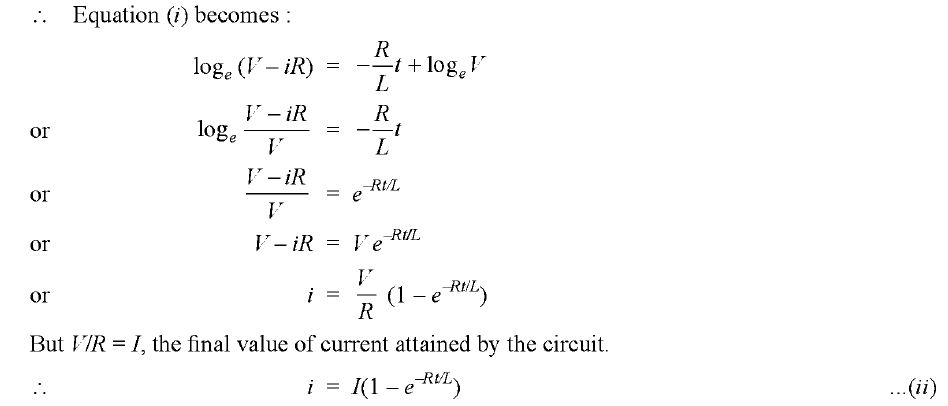
                                                                                           figure (b)

Eq. (ii) shows that rise of current follows an exponential law See Fig. (b). As t increases, the term e^-Rt/L gets smaller and current i in the circuit gets larger. Theoretically, the current will reach its final value I (= V/R) in an infinite time. However, practically it reaches this value in a short time.

Note. V = iR+ L di/dt

At the instant the switch is closed, i = O. V'= L di/dt

The initial rate of rise of current in an inductive circuit helps us in defining the time constant of the circuit